
Michael Gratton
Department of Mathematics
Harvey Mudd
College
Michael Gratton
 Presently at
Duke University (Mathematics)
Presently at
Duke University (Mathematics) Thesis: Mixing in Helical Pipes (PDF, 1.2M)
Advisor: Professor Andrew J. Bernoff
Awards:
- Chavin Prize for Undergraduate Thesis
- Honors in Humanities
- Graduated with Distinction
- Freshman Writing Prize
E-Mail: mgratton@math.duke.edu
Ciriculum Vitae: cv.pdf
Mixing in Helical Pipes
In everything from a coiled garden hose to fluid-based cooling systems, mixing in a spiralling pipe (like the one shown at the top left of this page) is very common. However, not much is known about how the tightness of the coils of the pipe affect the mixing. In our work, we simulated flow in a helical (spiralling) pipe with a computer model and examined how the pipe geometry changed the mixing.
In this model, the parameter lambda = torsion/curvature controls the tightness of the pipe's coils. Lambda = 0 represents a toroidal pipe (a doughnut shaped pipe, or a curved section). Increasing lambda straightens the pipe like streaching a coiled telephone cord. For lambda approaching infinity, the pipe becomes nearly straight.
Mixing in straight pipes is well-understood. Figure 1shows a side view of a straight pipe in which dye (simulated as the blue particles) is released in the center of the left end. The pressure driving the fluid through the pipe causes the dye to be swept out in a nosecone shape. Molecular diffusion causes the shape to "blur". Also plotted in the movie is the width of the dye cloud, sigma, measured in the down-the-pipe direction. When we speak of mixing, we want to know how sigma changes with time.
In Figure 2, dye is released at the bottom of a toroidal pipe (lambda = 0) and is swept upward. The curve of the pipe causes the fluid flow to form two rotating vorticies. In this figure, the pipe is shown as straight so the behavior is easier to see. In Figure 3, a line of dye starts at the bottom of a helical pipe (lambda = 0.1). Once again, the pipe is shown as straight. Notice that in addition to the vorticies seen in the toroidal pipe, the vorticies are actually rotating about each other. For larger values of lambda, the vorticie's behavior is difficult to discern (see Figure 4).
In our experiments, we release a circular wall of dye, following Lighthill (1966). Graphing sigma versus time shows three mixing behaviors, or regimes (see Figure 5). For small times, the pipe behaves as though it were straight regardless of the value of lambda, with sigma changing linearly with time as noted by Lighthill (1966). After some time, we notice that the dye still spreads linearly, but now lambda controlls the slope. Figure 6 shows the change in slope. Finally, for large times (t >> 1/D), we see self-similar diffusion.
These results can be verified using a technique known as streamline-averaging. Provided diffusivity is small and t is smaller than 1/D (where D is the diffusivity), a particle cannot escape from whatever streamline it began on. Sigma can be calculated by determning how much dye begins on a given streamline and the average speel of particles on that streamline down the pipe. Figure 7 shows a plot of slope versus lambda. The circles represent data from the numerical simulation and the line shows the streamline average data. Agreement is good, especially for large lambda.
More details can be found in the thesis linked above.
--mg
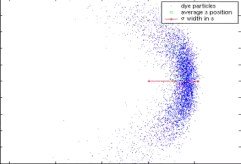
Figure 1: Pressure-driven pipe flow and the sigma measurement of mixing. Low quality mpeg (930k) or high quality avi (1M).

Figure 2: Point release of dye in a toroidal pipe. Low quality mpeg (320k) or high quality mpeg (920k).
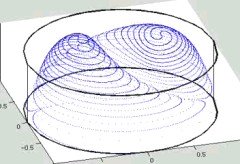
Figure 3: Line release of dye in a helical pipe (lambda = 0.1). Low quality mpeg (390k) or high quality mpeg (1.1M).

Figure 4: Line release of dye in a helical pipe with lambda = 1. Low quality mpeg (320k) or high quality mpeg (920k).
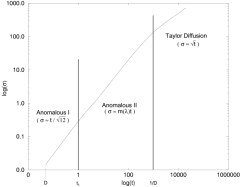
Figure 5: Log-log plot of sigma versus time for lambda = 1. High quality version.
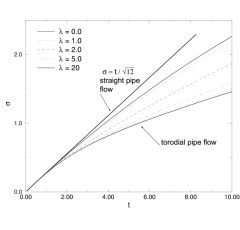
Figure 6: A plot of sigma versus time for various values of lambda shows the dependence of the mixing on geometry. High quality version.
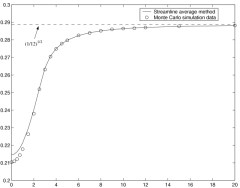
Figure 7: Speed of mixing versus lambda for moderate timescales. High quality version.
References
- W.R. Dean. Note on the motion of a fluid in a curved pipe. Phil. Mag, 4:208-223, 1927.
- M. Germano. On the effect of torsion on a helical pipe flow. J. Fluid Mech., 125:1-8, 1981.
- Scott W. Jones, Oran M. Thomas, and Hassan Aref. Chaotic advection by laminar flow in a twisted pipe. J. Fluid Mech., 209:335-357, 1989.
- Marco Latini and Andrew J. Bernoff. Transient anomalous diffusion in Poiseuille flow. J. Fluid Mech., 2001.
- M.J. Lighthill. Initial development of diffusion in Poiseuille flow. J. Inst. Maths Applics, 2:97-108, 1966.
- Joseph F. Lingevitch and Andrew J. Bernoff. Advection of a passive scalar by a vortex couple in the small-diffusion limit. J. Fluid Mech., 270:219-249, 1994.
- G.I. Taylor. Dispersion of soluable matter in solvent flowing slowly through a tube. Proc. R. Soc. Lond. A, 219:186-203, 1953.